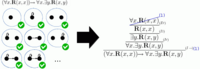
Gödel's completeness theorem

Imagine that you are playing with a big box of Legos. You want to build something, but you need to be sure that you have all the pieces you need. That's a bit like what Gödel's completeness theorem is all about!

The theorem is a fancy way of saying that if you have a set of rules or axioms (like the instructions for building something with Legos), then you can find a way to build any possible object (like a spaceship, a castle, or a dragon) using those rules.

Here's an example: let's say we have a bunch of building blocks that we can use to build different shapes. We have some rules for how the blocks can be put together – for instance, we might say that two blocks of the same color can't be next to each other. With these rules, we can build lots of different things – a house, a car, or a boat.

But what if we want to make sure that we can build any possible shape with these blocks and rules? Gödel's completeness theorem tells us that we can find a way to do that by adding a few more rules. These new rules might tell us how to make certain shapes, or how to put any two blocks together in a certain way.

So, in the end, Gödel's completeness theorem is all about making sure that we have everything we need to build any possible shape or object using a set of rules or axioms. It's like having all the pieces in your Lego kit, and knowing all the rules for how to put them together – you can build anything you can imagine!