Japanese theorem for concyclic polygons

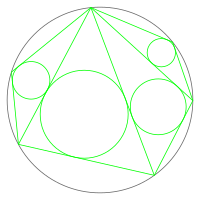
The Japanese theorem for concyclic polygons says that if you have a polygon with an odd number of sides and all of the vertices of the polygon lie on the same circle (like some kind of special bracelet that's perfectly round), then you can draw straight lines connecting the midpoints of the sides of the polygon and those lines will all meet at the center of the circle.

It's a little bit like having a carousel with animals on it - as long as there are an odd number of animals (like a lion, a zebra, and a giraffe), and they're all attached to the carousel in just the right way so they're all touching the same circle, there's a special point in the middle where you could put a really cool decoration, like a glittery star or something.

But, just like how not all carousels are the same, not all polygons work for this rule - it needs to be an odd number of sides and all the vertices have to be on the same circle. And just like how sometimes you have to adjust the animals on a carousel to make sure they're all riding securely, sometimes mathematicians have to tinker and tweak their polygons to make sure the theorem works.