Sinhc function

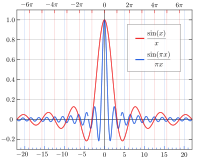
Okay, so imagine you're playing with a spring that's stretched out. When you pull the spring, it resists your pull and tries to return to its original shape. The sinhc function is kind of like that spring, in that it resists getting stretched out too much.

Now, the way the sinhc function actually works is a bit more complex than a simple spring. It's a type of mathematical function that takes in a number (let's call it x) and spits out another number. The output number is based on a special formula that involves the constant number "e" (which is a really important number in math).

The sinhc function is interesting because it looks kind of like the regular sin function (which also involves "e"), but with a "c" added in there. This "c" tells the sinhc function to resist getting stretched out too much. It's like a limit on how far the function can go, just like how a real spring can only stretch so far before it breaks.

So if you graphed the sinhc function, you'd see a curve that grows quickly at first but then levels off and stops growing so fast. This is kind of like how a spring might stretch really quickly at first but then slow down as it approaches its limit.

Overall, the sinhc function is a really cool mathematical tool that can be used to model all kinds of physical and abstract situations where there's a limit on how far something can go.