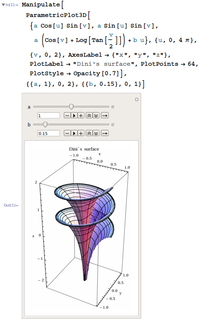
Dini's surface

Imagine you have a big lumpy ball, like a potato or an orange. If you look very closely at the surface of the ball, you'll see that it's not perfectly smooth - there are little bumps and dips all over it.

Scientists are really interested in studying these bumpy surfaces because they can tell us a lot about the properties of different materials. But it's hard to study them directly because they're so small and intricate.

That's where Dini's surface comes in. A mathematician named Ulisse Dini came up with a way to create a surface that is covered in little bumps and dips, just like the surface of our ball. But the surface he created is special because it has some really nice mathematical properties that make it easy to study and understand.

You can think of Dini's surface as a pretend ball that's covered in bumps and dips that are all the same size and shape. By studying this pretend ball, scientists can learn more about how bumps and dips work on real-life surfaces like rocks, metals, and even our own skin!