Filters in topology
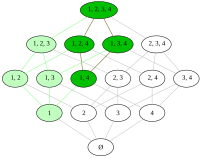
Okay kiddo, let's chat about filters in topology. Imagine you have a big pile of toys and you want to sort them out. You might first group together all the blue toys, then all the red toys, and so on. This is called a filter.
In topology, we're not sorting toys, but instead we're looking at how sets of points in a space relate to each other. Think of a space as a big playground with lots of points, like swings, slides, and monkey bars. A filter in topology is a way of grouping together some of those points based on certain rules.
One common rule involves neighborhoods. Imagine you're playing on the monkey bars, and you want to group together all the kids who are close to you. You might say, "Everyone who can reach this green bar is in my neighborhood." In topology, we can do the same thing. We might say, "All the points that have a neighborhood contained in this bigger set are in my filter."
Another way to think of filters is as a way of gradually zooming in on a space. Imagine you're looking at a map of your town. You might start by looking at the state or country, then zoom in to the city, and then to individual neighborhoods. In topology, we can do something similar by looking at larger and larger sets of points, and then gradually refining our filter to focus on smaller and smaller neighborhoods.
Filters can also tell us something about the structure of a space. For example, if a filter contains a certain point, then all the neighborhoods of that point are also in the filter. This means that if we make the neighborhoods smaller and smaller, we'll still get points in the filter.
Finally, filters can be used to define certain concepts in topology, like continuity and convergence. These are big words, but they basically mean that things in a space behave in a predictable and understandable way.
So filters in topology are a way of sorting and grouping together points in a space based on certain rules or neighborhoods. They can help us understand the structure of a space and the behavior of its points.
In topology, we're not sorting toys, but instead we're looking at how sets of points in a space relate to each other. Think of a space as a big playground with lots of points, like swings, slides, and monkey bars. A filter in topology is a way of grouping together some of those points based on certain rules.
One common rule involves neighborhoods. Imagine you're playing on the monkey bars, and you want to group together all the kids who are close to you. You might say, "Everyone who can reach this green bar is in my neighborhood." In topology, we can do the same thing. We might say, "All the points that have a neighborhood contained in this bigger set are in my filter."
Another way to think of filters is as a way of gradually zooming in on a space. Imagine you're looking at a map of your town. You might start by looking at the state or country, then zoom in to the city, and then to individual neighborhoods. In topology, we can do something similar by looking at larger and larger sets of points, and then gradually refining our filter to focus on smaller and smaller neighborhoods.
Filters can also tell us something about the structure of a space. For example, if a filter contains a certain point, then all the neighborhoods of that point are also in the filter. This means that if we make the neighborhoods smaller and smaller, we'll still get points in the filter.
Finally, filters can be used to define certain concepts in topology, like continuity and convergence. These are big words, but they basically mean that things in a space behave in a predictable and understandable way.
So filters in topology are a way of sorting and grouping together points in a space based on certain rules or neighborhoods. They can help us understand the structure of a space and the behavior of its points.
Related topics others have asked about:
