Group of rational points on the unit circle

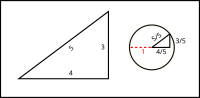
Imagine a circle that's completely round like a pancake, kinda like a cookie but it has no edges. This circle is called the unit circle and it has a special size, like a special toy that dad buys you on your birthday. Now, let's say you have some friends who are also on this same circle, and they are all standing in different places on the circle.

Now think about something called "rational numbers." These are numbers that can be made by dividing one whole number by another. For example, cutting an apple in half is like dividing 1 apple into 2 equal parts, so half is the rational number 1/2.

So, we are looking for a group of points that are both on the unit circle and are also rational numbers. Some of your friends may be standing on points that are rational numbers, like maybe your friend is on the point (1, 0) which is the same as saying they are on the number 1. Other friends might be standing on points that aren't rational numbers, like maybe your friend is on the point (sqrt(2)/2 , sqrt(2)/2) which is not a rational number because you can't divide whole numbers to get that.

So, the group of rational points on the unit circle are just the points on the circle that have rational numbers as their coordinates, and they are your friends who are jumping around on the circle. They might be hard to find, but if you look hard enough, you'll find them!