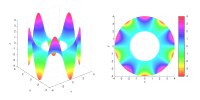
Harmonic function

Okay kiddo, have you ever played with a yo-yo? It goes up and down, up and down, right? Imagine that the yo-yo goes up and down in a very special way. It always goes the same distance up and the same distance down. And it never stops, it keeps going up and down forever.

Now, if we were to draw a line that shows where the yo-yo is at any given time, what do you think that line would look like? Would it be a straight line, a wiggly one, or a curve?

Well, it turns out that the line would be a curve, and it would also be a very special curve called a "sine curve." And if we were to look at this curve on a graph, it would look like a wave with peaks and valleys that repeat over and over.

Now, let's call this yo-yo the "harmonic oscillator," and let's say that it's always moving in response to some force or energy that's acting on it. And just like the yo-yo moves up and down in a regular way, the energy that's acting on it moves in a regular way too.

This energy is what we call a "harmonic function." It's a special kind of function that repeats itself over and over, just like the yo-yo's motion. And just like the yo-yo's motion produces a sine curve, the harmonic function produces a wave-like pattern on a graph.

So, to sum it up, a harmonic function is like a yo-yo that goes up and down in a regular way, and the line that shows where the yo-yo is at any given time is a special curve called a sine curve. This special curve is produced by a repeated energy pattern that we call a harmonic function.