Integration (calculus)

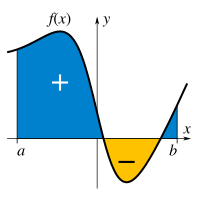
Integration in calculus is like putting together a puzzle. Imagine you have a big puzzle with lots of tiny pieces. Let's say you want to know how much area is covered by those pieces. You could use integration to add up all the tiny areas to get the total area.

First, you break the area into small rectangles, which is like breaking the puzzle pieces into small groups. Then, you find the area of each rectangle by multiplying its height and width. That's like figuring out the size of each puzzle piece.

Once you know the area of each rectangle, you add them all up. This gives you an estimate of the total area. However, the estimate might not be very accurate if the rectangles are too big or if there are gaps between them.

To get a better estimate, you break the area into even smaller pieces. So instead of using rectangles, you could use triangles, circles, or other shapes. The smaller the pieces, the more accurate the estimate will be.

At some point, if you keep breaking the area into smaller and smaller pieces, you will get an exact answer for the total area. That's what integration does! It adds up tiny pieces of an area, and as those tiny pieces get infinitely small, you get the exact answer.

Integration is used in many fields, like engineering, physics, and economics. For example, integration can help you find the volume of a shape or the amount of work done by a force. It's like a magic tool that helps you solve all kinds of puzzles!