Brachistochrone

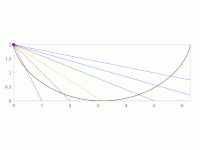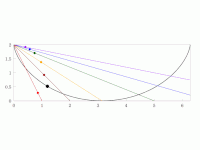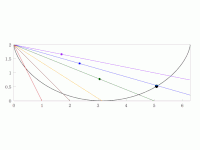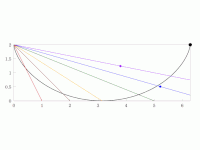
Have you ever played with a ball, and you wanted to make it go from one point to another? What is the quickest way to make the ball roll from one point to another? A brachistochrone curve is a cool mathematical shape that shows you the fastest way an object can travel between two points without being pulled by anything or pushed. Think of it like a playground slide - you want to go from the top to the bottom as quickly as possible, but you can't use your feet or hands to push yourself down.

Now, what makes the brachistochrone curve special is that it isn't a straight line. Instead, it's a curved line that starts off steep and gradually becomes less so. You might think that the fastest route from point A to point B would be a straight line, but that's not true. The brachistochrone curve is the shortest path between two points, which also means it's the fastest. So, when you roll a ball down the brachistochrone curve, it gets there faster than it would have if you rolled it down a straight line or a different type of curve.

Scientists and mathematicians have been studying the brachistochrone curve for centuries. It's not just about rolling balls, either. They use these curves to figure out how things like roller coasters and airplanes move. By understanding the brachistochrone curve, they can design faster, more efficient machines that save time, energy, and money. So the next time you see a spiral stair or a slide that has a fantastic curve, remember that you're looking at a real-life example of a brachistochrone curve!