Japanese theorem for cyclic quadrilaterals

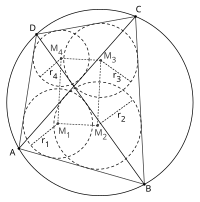
Okay kiddo, there is something called the "Japanese Theorem" which helps us to find the area of a "cyclic quadrilateral".

Now, a "cyclic quadrilateral" is like a four-sided shape with all four corners on a circle. Kind of like drawing a square or rectangle, but instead of the corners being at right angles, they are on a circle.

So, the Japanese Theorem tells us that if we have a cyclic quadrilateral and we draw the diagonals (the lines that connect opposite corners), then we can find the area of the shape by using a special formula:

Area = (Diagonal 1) x (Diagonal 2) x sin(θ) / 2

Now, don't worry about that "sin(θ)" part, that's just a fancy way of saying we need to use some math to find it. But basically, we just need to know the length of the two diagonals, and then we can use this formula to find the area.

So, why is this helpful? Well, sometimes we need to find the area of unusual shapes, and a cyclic quadrilateral is one example. By using the Japanese Theorem, we can solve this problem and figure out the area using just some basic math skills.

And there you have it, the Japanese Theorem for Cyclic Quadrilaterals explained like you're 5!