Projective scheme

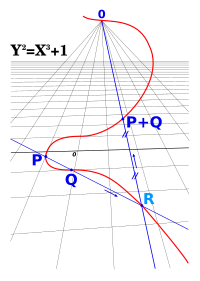
A projective scheme is like a special kind of picture that shows points that are related to each other in a certain way. Think of a coloring book with different shapes, like circles and squares. Usually, we color these shapes and leave the rest of the page blank. But with a projective scheme, we color the whole page, and the colors tell us how the shapes are related to each other.

Imagine we have a bunch of points in space, and we want to see how they are connected. We can draw lines between them, but we might miss some important connections if we only focus on straight lines. A projective scheme helps us see all the connections by looking at how things change when we move around in space.

To make a projective scheme, we imagine a big, imaginary space that contains all the points we're interested in, even the ones that seem far away or don't seem to belong together. This imaginary space is like a giant playground where we can move the points around and see what happens.

Then we draw a special type of shape in this imaginary space called a hyperplane. A hyperplane is like a flat surface that goes through all the points we're looking at. This surface is so big that it stretches out to infinity and includes every point we're interested in.

Once we have this hyperplane, we can look at how it intersects with the points we're trying to understand. Sometimes it will slice a point into two pieces, or it might make two far-apart points look like they're connected. As we move the hyperplane around, we see different pictures of how the points are related to each other.

If this all sounds very abstract and hard to visualize, that's because it is! But projective schemes are used by mathematicians to study some of the most exciting and fundamental questions in geometry and algebra. By looking at how points behave under different transformations, we gain new insights into their properties and can solve puzzles that would be impossible to figure out in any other way.