Logistic differential equation

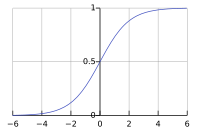
Okay kiddo, let’s try to understand what a logistic differential equation means.

Imagine you have a toy room full of toys. At first, you only have a few toys, but as time goes on, your toy collection keeps growing. At some point, your room will get too full, and you won’t have space for any more toys.

The logistic differential equation is like your toy room. It shows how something starts small and grows over time but eventually reaches its limit. This equation is often used in population growth models, such as estimating how many fish can live in a pond.

In simpler words, the logistic differential equation is like a math equation that shows how a population grows and eventually reaches its maximum limit. It helps us predict how many living things can coexist in an area without running out of resources.

So, just like your toy room, there is a limit to how much can fit in one space, and the logistic differential equation helps us understand that limit. Does that make sense, kiddo?